Employed by the ancient Egyptians, Greeks, and Mesopotamians, the earliest calculating devices were systems of writing that used shorthand to denote specific and often large quantities. These written forms differed between cultures but usually involved groups of lines representing single units, with modified characters for intervals of five or ten.
Counting sticks, knots, and tally sticks - with values denoted by specific notches - were common forms of counting and numerical record-keeping throughout the world. These systems, along with the use of Roman numerals, persisted through the Renaissance, as many were hesitant to adopt the Hindu-Arabic numerals used today out of concern for accuracy and the potential for forgery.
The abacus is perhaps the most well known pre-modern calculating device, and is often associated with the wire-and-bead devices that originated in the Middle East. While its true origins remain debatable, the word abacus would have referred to an ancient practice of moving pebbles ('calculi') along lines written in sand.
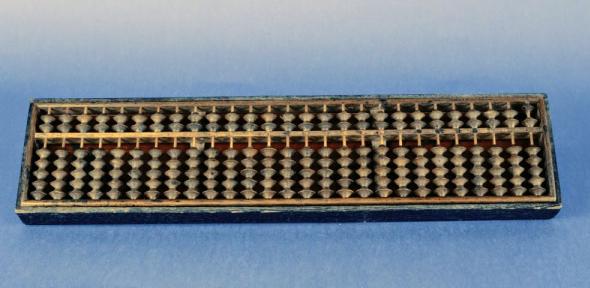
A common abacus today is the Japanese 'soroban', which has one 'heavenly' bead per wire representing 5, and four 'earthly' beads representing 1 each. This is a simplification of the Chinese 'Suanpan', in which more beads per wire can accommodate other decimal systems such as duodecimal (i.e. base 12, rather than base 10) (Image 1, above).
Pure mathematics has its own history alongside that of counting. The origins of geometry, for example, stretch back to Ancient Greece, and Euclid's Elements, first compiled around 300 BCE, would become, in various forms, the standard mathematical textbook for nearly two millennia (Image 2).
Read more: how models were used to aid the teaching of Euclid's Elements.
The Early Modern period
The Early Modern period in Europe (roughly from the 15th to the 18th centuries) saw the development of many new calculating tools, as well as the revival and adaptation of several from classical and Middle Eastern culture. For instance the astrolabe, invented in antiquity and developed during the Islamic Golden Age (roughly the 7th to the 15th centuries), was a multi-purpose tool used to measure the heights of buildings and record planetary motions.
Slides, cranks, and dials
Most importantly, after 1400 CE new tools and techniques were developed for commerce, exploration, and natural philosophy, often serving multiple purposes. From the 17th century, the slide rule, for instance, became the most commonly used calculating device for nearly three hundred years. Beginning as a 'line of numbers' arranged on wood, paper, or brass, rulers attached to one another were used to align points along different scales to perform arithmetic and convert units.
